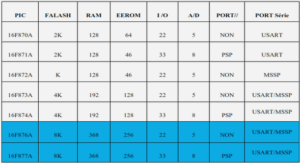
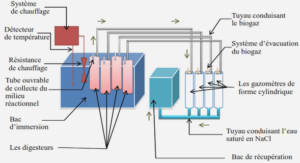
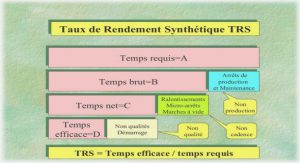
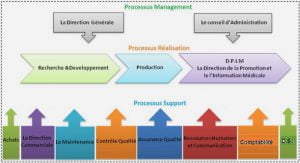
THEORETICAL FOUNDATION S 

THEORETICAL FOUNDATION S 

REVIEW O F PREVIOUS RESEARC H

Geometri c inspection o f flexible parts

Traditional toleranc e analysi s methods , suc h a s Root Sum Square method an d Monte Carlo simulation (Crevelin g (1997)) , ar e no t applicabl e t o complian t part s suc h a s shee t meta l assemblies becaus e o f possible part deformations durin g th e assembl y process. Ove r the past years, differen t method s hav e bee n presente d t o predic t dimensiona l variatio n o n flexible parts, especiall y o n shee t meta l assemblies . Mos t o f th e method s ar e base d o n th e Finite Element Method. Li u an d H u (1997 ) presente d a mode l t o analyz e th e effec t o f componen t deviations and assembly spring-bac k o n assembly variatio n b y applying linea r mechanics and statistics. Usin g FEM , the y constructe d a sensitivit y matri x fo r complian t part s o f comple x shapes. Th e sensitivit y matri x establishe d a linea r relationshi p betwee n th e incomin g par t deviation an d th e outpu t assembl y deviation . Chan g an d Gossar d (1997 ) presente d th e transformation vector s t o describ e variatio n an d displacemen t o f features. The y modele d th e parts and tooling a s groups of features. Th e method represente d th e interaction betwee n parts and tooling by contac t chains. A contact modeling algorithm implemente d int o the Method o f Influence Coefficien t t o prevent penetration s betwee n parts has been presented b y Dahlstro m and Lindkvist (2007).
Non-contact 3 D digitizin g system s expose d a ne w horizo n i n geometri c inspectio n o f bot h rigid an d nonrigi d parts , becaus e the y delive r muc h mor e dat a tha n mechanica l probes , i n a shorter time . Weckenman n an d Gabbi a (2005 ) propose d a measurin g metho d usin g virtua l distortion compensation . Fring e projectio n system s ar e suitabl e fo r th e fast an d contac t fre e measurement o f parts without clamping. They used the measurement resul t to extract feature s of th e objec t lik e hole s o r edges . Som e o f thes e wer e relevan t fo r th e assembl y process ; others wer e subjec t t o furthe r inspection . Fro m th e informatio n abou t th e transformatio n o f the assembl y feature s fro m thei r actua l t o thei r nomina l position , virtua l distortio n compensation wa s use d t o calculat e featur e parameter s o f th e distortio n compensate d shape Their metho d wa s no t completel y automate d becaus e th e suggeste d metho d neede d som e human challenge s t o identif y th e correlatio n betwee n som e specia l point s lik e hole s an d assembly joint positions. These le d the controller to find the boundary conditions o f the FEM problem. Besides , transformin g th e poin t clou d t o a compute r aide d analyzabl e mode l i s a very time-consumin g process . I t seem s tha t thi s metho d i s no t suitabl e fo r reall y flexible parts becaus e the y hav e no t considere d th e effec t o f gravit y an d th e 3 D situatio n whic h th e part has scarmed. The concep t o f th e Small Displacement Torsor (SDT ) ha s bee n develope d b y Bourde t an d Clement (1976 ) to solve the general problem of the fit of a geometrical surfac e mode l to a set of points using rigid bod y movements. Lartigue , Thiebaut e t al. (2006) too k advantag e o f the possibilities offere d b y voxe l representatio n an d SD T methods fo r dimensiona l metrolog y o f flexible parts . Thi s time , the y considere d th e effec t o f gravit y an d spatia l situatio n o f a scanned part . Thi s metho d i s fundamentall y base d o n findin g th e correlatio n betwee n th e cloud o f all measure d point s an d CA D meshe d data . I n fact , th e SD T is mor e suitabl e fo r a small deformation . Mor e accurat e results ca n eve n b e achieve d i f one considers th e effec t o f material flexibility.
Abenhaim, Taha n e t al . (2009 ) develope d iterative displacement inspection (IDI ) whic h smoothly deforme d th e CA D mes h dat a unti l matche d t o th e rang e data . Thei r metho d wa s
based o n optima l ste p nonrigi d IC P algorithm s (Amberg , Romdhan i e t al . (2007)) . Th e proposed ID I metho d wa s full o f limitations. Their metho d wa s no t teste d i n non-continuou s areas suc h a s holes, an d th e poin t clou d neede d t o b e dens e enoug h becaus e th e method’ s similarity measur e wa s only based o n the nearest distanc e calculation. Th e majo r flaw o f this method wa s hidde n i n th e fac t tha t i t strongl y depende d o n findin g som e trial s an d prio r flexibility parameter s whic h coul d var y dependin g o n thickness . Furthermore , th e metho d supposes tha t th e boundar y o f measured parts is without defec t s o the metho d i s not suitabl e in th e cas e o f shrinkage . Th e mentione d drawback s caus e th e ID I t o b e ineffectiv e i n rea l engineering applications.  Including part compliance wit h intrinsic geometry of surface i n metrolog y o f free-for m surfaces, is an area of research pioneered i n this thesis.

Rigi d and nonrigid surfac e registratio n

Parallel t o mechanica l engineer s bu t i n differen t fields lik e Computer science. Biomedical engineering an d Pattern recognition, ton s o f researc h ha s bee n don e o n Rigi d an d Nonrigi d Registration an d deformabl e surfac e compariso n domains . Bes l an d McKa y (1992 ) developed a n iterativ e metho d fo r th e rigi d registratio n o f 3 D shapes . Th e ide a behin d th e iterative closest poin t (ICP ) algorithms is simple: give n two surfaces, Xand Y, find the rigi d transformation (R, t), s o tha t th e transformed surfac e Y’= RY + / is as ‘close ‘ a s possible t o X. ‘Closeness ‘ i s expressed i n terms o f some surface-to-surfac e distanc e d (RY + /, X). Mor e precisely, ICP can be formulated a s a minimization problem : d ,cp(X. Y) = min «,, d{RY +t,X) (1.1 ) This algorith m differ s i n th e choic e o f th e surface-to-surfac e distanc e d fY’ X) an d th e numerical metho d fo r solvin g th e minimizatio n problem . Th e IC P algorith m i s on e o f th e common technique s fo r refinemen t o f partia l 3 D surface s (o r models ) an d man y varian t techniques have been investigated . However, searchin g the closest poin t i n the ICP algorithm is a computationall y expensiv e task . I n orde r t o accelerat e th e spee d o f closes t poin t searching, some search technique s ar e commonly employed . Many variants of ICP have bee n proposed, affectin g al l phases of the algorithm – from th e selection an d matching of points, to the minimizatio n strategy . Th e correspondenc e betwee n point s i s usuall y performe d b y a nearest-neighbour searc h usin g a k-d tree structur e fo r optimizatio n (Bentle y (1975)) . The k – d tre e i s a spatia l dat a structur e originall y propose d t o allo w efficien t searc h o n orthogona l range querie s an d neares t neighbou r querie s (Bentle y (1975)) . Greenspa n an d Godi n (2001 ) proposed a significan t improvemen t i n th e neares t neighbou r querie s b y usin g correspondences o f previou s iteration s o f th e IC P an d searchin g onl y i n thei r smal l neighbourhood t o updat e th e correspondences . Anothe r importan t strateg y t o spee d u p th e  registration proces s use s samplin g technique s t o reduc e th e numbe r o f points i n th e view s (Rusinkiewicz an d Levo y (2001)). Myronenko, Son g e t al . (2007 ) introduce d a probabilisti c metho d fo r rigid , affine , an d nonrigid poin t se t registration , calle d th e Coherent Point Drift algorithm. The y considere d the alignmen t o f tw o poin t set s a s th e probabilit y densit y estimation , wher e on e poin t se t represents th e Gaussia n Mixtur e Mode l centroid , an d th e othe r represent s th e dat a point . They iterativel y fitte d th e GM M centroid s b y maximizin g th e likelihoo d an d foun d th e posterior probabilitie s o f centroids , whic h provid e th e correspondenc e probability . Th e method base d o n forcin g th e GM M centroid s t o mov e coherentl y a s a group , preserve d th e topological structur e o f the point sets. Schwartz, Sha w e t al . (1989 ) wer e th e first tha t use d Multidimensional Scaling (MDS ) methods t o flatten th e curve d convolute d surface s o f th e brai n i n orde r t o stud y functiona l architectures an d th e neura l map s embedde d i n them . Fo r some , thei r wor k wa s a breakthrough i n which surfac e geometr y wa s translated int o a plane. But the plane restrictio n introduced deformation s tha t actuall y prevente d th e proper matchin g o f convolute d surfaces .
This problem can be solved if higher dimensions of the embedding spac e ar e considered . The Fast marching method wa s introduce d b y Sethia n (1996 ) a s a computationally efficien t solution t o Eikonal equations o n flat domains. A related metho d wa s presente d b y Tsitsikli s (1995). Th e fas t marchin g metho d wa s extende d t o triangulate d surface s b y Kimme l an d Sethian (1998). The extende d metho d solve d Eikona l equation s o n flat rectangula r o r curve d triangulated domains. Elbaz and Kimme l (2003 ) presented a blend of topology an d statistica l methods, to introduc e the concept of Invariant signature fo r surfaces. Their metho d wa s based o n fast marchin g o n triangulated domai n algorith m followe d b y MD S technique . The y hav e practicall y transformed th e proble m o f matchin g isometric-nonrigi d surface s int o th e proble m o f matching o f rigid surfaces. Usin g MDS, they embedded surface s X and Y into some commo n  embedding spac e Z calle d Canonical form an d the n measure d th e similaritie s usin g th e Hausdorff’ distance. Thei r metho d i s strongl y base d o n th e Kimme l an d Sethia n (1998 ) method i n computing the geodesic distanc e on triangular meshes.

Table des matières

INTRODUCTION 1 
CHAPTER 1 REVIEW O F PREVIOUS RESEARC H 1
1.1 Geometri c inspectio n o f flexible parts
1.2 Rigi d and nonrigid surfac e registratio n
CHAPTER 2 THEORETICAL FOUNDATION S 
2.1 Metri c space s
2.2 Poin t clouds techniques
2.2.1 Smoothin g o f noisy data
2.2.2 Poin t cloud samplin g
2.2.3 Fas t marching method
2.3 Isometri c embedding
2.4 Generalize d multidimensiona l scalin g
CHAPTER 3 NONRIGID GEOMETRIC INSPECTIO N 
3.1 Geometr y o f flexible parts
3.2 Identificatio n o f geometrical deviatio n
3.3 Ne w definition fo r maximum geometri c deviation
3.4 Numerica l inspectio n fixtur e
3.5 Generalize d numerica l inspection fixture
CHAPTER4 RESULTS 
4.1 Geometri c inspectio n i n absence of spring-back
4.2 Inspectio n results with GNIF
CONCLUSION 5 
Comparison o f methods
Limitations of GNIF
Contributions
RECOMMENDATIONS
ANNEX I PUBLICATION S
ANNEX II SMACO F algorithm
ANNEX III Hausdorf f distanc e algorithm
ANNEX I V GLOSSAR Y
REFERENCES

Télécharger le rapport complet