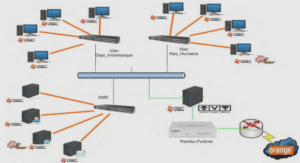
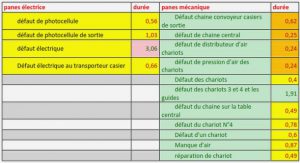
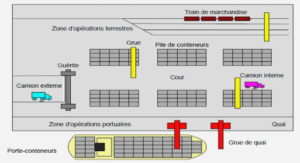
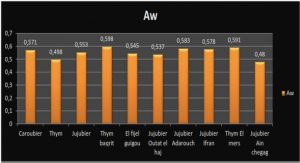
History of damage models
While rupture mechanics aims at the description of a macroscopic crack that goes through a structure whose material is sound, damage mechanics aims at describing the phenomenon that lead to the apparition of a macroscopic crack. The state of damage characterises the state of a material between the perfectly sound material exempt of any micro defects such as micro cracks or micro cavities, and the existence of a crack at a mesoscale. In the framework of 1 continuum mechanics, damage models use an internal variable to describe the deterioration of the material via the quantication of defects present in the material. At the macroscale, this deterioration can be observed through its eects on macroscopic properties of the material: it can decrease the elasticity modulus, the yield stress (when coupled to plasticity), the density, etc. An homogenisation operation is introduced in order to quantify the presence of micro defects in the material and to dene an average of the stress and strain elds. Let us consider a solid in which a damaged representative volume element (RVE) was isolated, and whose sound section is noted δS and damaged section δSD.Many phenomenological damage laws have been introduced in the past. One of the rst was Kachanov’s visco-plastic damage law, introduced in 1958 [31], in which he introduced a macroscopic damage variable to describe the creep. His pioneering work was soon followed and the theory of continuum damage mechanics (CDM) thoroughly developed in the last decades. It is well described in the book of Lemaitre [37] or Lemaitre and Desmorat [39]. Nowadays, many damage laws exist in the literature, and can be classied into two main families: the local and non local damage laws. Local damage laws state that the damage at one point of the structure does not depend on the damage on the surrounding points. These models have been proven to be unsuitable to model the damage evolution of a structure, for various reasons. First, one can show that it is possible, using these models, to break a structure, i.e. reach a damage value that is maximum, without any cost of energy. Indeed, the damage band can be taken as thin as one wants, and when it reduces to zero, the dissipated energy which is the integral of the dissipated density energy over the domain also reduces to zero. This a a rst reason that demonstrates that local models do not work well. Moreover, it has been shown that they induce a very strong dependency to the mesh: the damage band width is equal to the mesh element size. Finally, demonstrations of the instability of the solutions of the damage evolution have also been made. Thus, there are physical, numerical and mathematical arguments that caution us not to use local damage models. To alleviate all these faults, non local damage models have been introduced. A review of these models can be read in [8]. There are two main schools regarding the formulation of the (a) Sharp crack (b) Regularisation with `/2 (c) Regularisation with whose width depends on the internal length `. non locality of the damage. The rst deals with the study of non local models of integral type. In these models, the constitutive law of a material at a given point contains terms that average a state variable, or a thermodynamic potential on a given vicinity of the considered point. The second group introduces gradient terms of state variables or thermodynamic forces. The common point of these two dierent approaches lies in the introduction of a characteristic length or internal length, that controls the width of the damaged zone. We will focus mainly on the non local damage models of gradient type, called « gradient damage models », and sometimes referred to in the literature as « phase eld models ». The introduction of a spatial gradient of the damage variable regularises the displacement discontinuity: the sharp interface of the crack which induces a jump of the displacement across the two lips of the crack is now replaced by a smeared zone of nite width where the damage is continuous, as can be seen on Figure I.1. The localisation of damage is also penalised: if the damage localises over a very thin band, the gradient is therefore very high and the dissipated energy can not be zero any more. The construction of these gradient damage models, introduced in 1998 by Francfort and Marigo [18] is the object of the next part
Modelling ingredients
To construct a damage model, three elements are necessary:
i) A damage parameter: here, it is a scalar (we are working with isotropic materials) variable, α, that takes its values in an interval [0, αM[. The value zero corresponds to a perfectly sound material while the value αM, which can be nite or innite, depending on the model, corresponds to the nal stage of damage in the structure.
ii) The dependency of the stress-strain relation to the damage parameter: for a xed value of α, the material has a linear elastic behaviour. It progressively loses rigidity when α grows: its elasticity is characterised by the relation α 7→ E(α) between the stiness tensor and the damage parameter.
iii) An evolution law of α.
Rheological models and energy density
The connection between stress and strain for a viscoelastic material can be set through the use of rheological models. In the following, we will focus on the Maxwell and the PoyntingThomson model, shown respectively on Figures II.12a and II.10b. The Maxwell model is the association in series of an elastic spring and a viscous dashpot, which, in the Poynting model, is replaced by a Kelvin-Voigt element. Note that in small deformation, Zener and Poynting-Thomson have similar responses, and thus are equivalent. The energy density associated to these models is hence the sum of the elastic energy of the springs and the dissipated energy in the dashpot. The total deformation is decomposed as the sum of an elastic and an inelastic (viscous) energy.
Rupture energy
The rupture energy is the integral over the damage band of the dissipated energy when a crack has appeared. For the Maxwell model, the damage width band 2d is the same as for linear elastic material. Moreover, when a crack has developed in the bar, there remains only the dissipated energy, and the viscous energy as well as the elastic energy do not play any role. Consequently, the rupture energy Gc is the same for a Maxwell material or a linear elastic material. For the Poynting-Thomson model, we have seen previously that the damage width band takes values that are always superior to the values of the Maxwell model. Since the rupture energy is the integral over the damaged region of the damage dissipated energy, it can only be greater or equal to the dissipated energy obtained with the Maxwell model.
Zener model
In the case of the Zener model, the elastic potential is the sum of two potentials: one is written with respect to the total deformation, to take into account the energy of the main spring, and the other is written with respect to the elastic deformation. Unlike small deformation, where the Zener and Poynting-Thomson model exhibit the same viscoelastic responses, there is a difference in the responses of these two models in large deformation. We use here the Zener model because it is a particular case of the generalized Maxwell model (with one branch) commonly used to model viscoelastic behaviours. The elastic potentials are again those of Ciarlet-Geymonat in 1D.
|
Table des matières
I Introduction
I.1 History of damage models
I.2 Construction of damage gradient models
I.2.1 Non regularised models
I.2.1.a Modelling ingredients
I.2.1.b Evolution law of α
I.2.1.c Softening and hardening behaviours
I.2.1.d Energy construction and stability
I.2.1.e Variational formulation of the evolution problem
I.2.2 Regularised model
I.2.2.a Why a regularisation?
I.2.2.b Irreversibility, stability, energy balance
I.2.2.c Localisation on a 1D bar
I.2.2.d Strength of the model
I.2.3 Numerical implementation
I.3 Notations
I.4 Project background and organization of the work
II Unidimensional study of gradient damage models for viscoelastic materials
II.1 Damage and viscoelasticity
II.1.1 Energy density, first order stability and energy balance in 3D
II.1.1.a Rheological models and energy density .
II.1.1.b First order stability
II.1.1.c Energy balance
II.1.2 Homogeneous damage
II.1.2.a Viscoelastic phase
II.1.2.b Damaging phase
II.1.3 Damage localisation
II.1.3.a Damage profiles
II.1.3.b Rupture energy
II.1.4 Numerical implementation
II.2 Damage and hyperelasticity
II.2.1 Unidimensional hyperelastic potential
II.2.2 Homogeneous damage evolution in a 1D hyperelastic bar
II.2.3 Localised damage evolution in a 1D hyperelastic bar
II.3 Damage and hyper-viscoelasticity
II.3.1 Maxwell model
II.3.1.a Energy
II.3.1.b First order stability
II.3.1.c Hyper-viscoelastic phase
II.3.1.d Homogeneous damage
II.3.1.e Damage localisation
II.3.1.f Numerical implementation
II.3.2 Zener model
II.3.3 Conclusion
III Numerical study of damage gradient models for large deformation
III.1 Numerical implementation
III.1.1 Recall: displacement problem in large deformation
III.1.1.a Choice of the hyperelastic law
III.1.1.b Variational formulations
III.1.2 Damage problem
III.1.3 Remark: implementation with the FEniCS library
III.2 2D simulations
III.2.1 General considerations
III.2.2 Particular case of a 2D plate under uni-axial traction
III.3 3D simulations
III.4 Conclusion
IV Damage initiation in zones of high hydrostatic pressure
IV.1 Motivations
IV.1.1 Take micro defects into account
IV.1.2 Properties of the desired model
IV.2 Inextensible 1D bar
IV.2.1 Analytical study
IV.2.1.a Homogeneous response
IV.2.1.b Localised response
IV.2.1.c Mixed response
IV.2.1.d Energies
IV.2.2 Numerical implementation
IV.3 Extension to higher dimensions
IV.3.1 Numerical implementation
IV.3.2 2D plate under uni-axial traction
IV.3.2.a Analytical developments
IV.3.2.b Numerical application
IV.3.3 Test inducing bi-axiality
IV.3.3.a Geometry
IV.3.3.b Results
IV.4 Comparison with experiments
IV.5 Large deformation
IV.6 Conclusion
V Cavitation phenomenon
V.1 Motivations and state of the art
V.2 Hyperelastic bifurcation
V.2.1 Equilibrium equation
V.2.2 Stability of the equilibrium equation
V.2.2.a Second derivative of the total energy
V.2.2.b Stability of the solution f0 for Ri = 0
V.3 Damage and cavitation
V.3.1 Comparison of critical loads
V.3.2 2D numerical tests
V.4 Conclusion
Conclusion
Bibliography
![]() Télécharger le rapport complet
Télécharger le rapport complet