Relevance of absolute luminosity measurements
For most of the measurements foreseen by the LHC experiments [13] a good understanding of the hard processes occurring in hadron collisions plays a crucial role. These processes are described by perturbative quantum chromodynamics (QCD), whose predictions are in agreement with the measurements performed at the Tevatron [14].
From the theory side, the factorisation theorem [15] provides the possibility to calculate a wide variety of hard-scattering inclusive and differential cross-sections in hadron-hadron collisions. The theoretical results for several benchmark hard-scattering processes can be seen in Fig. 1.1. In an experiment, the absolute cross-section for a certain process can be measured from the rate at which this process occurs and the luminosity (see Eq. (1.1)). Therefore, a precise knowledge of the absolute luminosity is necessary for an accurate comparison between theory and experiment. Given the available event statistics at the LHC, the measurements ✦ have the potential to further constraint the theoretical models (e.g. the models describing the proton structure) and the precision of the luminosity determination should not be a limiting factor.
The physics processes occurring in high-energy colliders are dominated by the strong interactions and, naturally, QCD provides their description. The hadron scattering processes can be classified as soft or hard. The soft processes include elastic and diffractive interactions and play an important role in the calculations related to the total cross-section and the underlying event. The soft QCD processes are dominated by non-perturbative QCD effects which are still difficult to calculate. On the other hand, the hard QCD interactions involve a large momentum exchange and can be calculated with good precision using perturbation theory [14]. Typical hard scattering processes are Drell-Yan (quark-antiquark annihilation producing a lepton pair with large invariant mass; the process can be mediated by a virtual photon or a W or Z boson) and the heavy-quark, Higgs boson or high transverse momentum jet production processes. These processes play central role in the physics programme of the LHC experiments.
The essential condition for the application of perturbative QCD for calculations of hard scattering processes of hadrons is the possibility to separate (factorise) the interaction between the partons of the two incoming hadrons from the interactions of the partons inside each of the hadrons. The viability of such an assumption and the domains of its applicability have been formulated in several factorisation theorems [15]. As the name suggests, the factorisation theorem states that in hard hadron-hadron scattering, where the partons of the incoming hadrons interact at short distance (i.e. at large momentum transfer Q2 ), we can separate the sensitivity to dynamics at different scales. Almost all applications of perturbative QCD use factorisation properties of some kind.
Parton distribution functions
The proton PDFs fi(x, Q2 ) describe the probability for finding partons (quarks and gluons) inside the proton that carry certain fraction of its momentum. The dependence of the PDFs on the momentum transferred in the scattering process, Q2 , is described by the DGLAP equation § [17]. The proton PDFs are universal in the sense that they do not depend on the exact nature of the scattering process. For example, the proton structure obtained from fits to deep-inelastic scattering data is the same as the one obtained in fixed target experiments or in hadron colliders. However, as will be detailed later, different experiments access different regions in the (x, Q2 ) plane and therefore the most complete description of the proton structure is possible only by combining the available experimental data. Currently a number of groups produce publicly available PDFs using different data-sets and analysis frameworks. Three of them, namely MSTW [16], CTEQ [18] and NNPDF [19], provide global PDFs obtained from global fits of data from HERA, Tevatron and fixed target experiments.
Calculation of partonic cross-section
The hard-scattering partonic cross-sections σˆij→X, which appear in Eq. (1.2) are calculated in the framework of perturbative QCD. The standard approach is to express the scattering amplitude for a given process as a series expansion in powers of the gauge coupling constant αs. The terms in the perturbative expansion can be represented with the help of Feynman diagrams, where their physical meaning becomes clear. The number of vertices in each Feynman diagram is related to the order of the coupling constant in the perturbative expansion.
Naturally, the calculation of the terms corresponding to each order of the coupling constant is not possible and therefore in QCD calculations approximations need to be made. A leading order (LO) calculation of a certain process involves only the terms in the perturbative expansion which contain the lowest possible power of the coupling constant. In many cases LO calculations are sufficient to describe principal features of the process under study and provide the first estimate of its cross-section. Expressions for the LO cross-section of many processes are widely available in the literature [20]. Higher order calculations include the contributions from more complicated Feynman diagrams, which involve larger number of interaction vertices. For example, a next-to-leading order (NLO) calculation includes all terms to order αs compared to the LO term. Higher-order calculations account for extra partonic processes that are possible only beyond the LO and therefore provide more precise estimates of the related quantities. The higher order QCD calculations offer significant challenges due to infrared divergences occurring from real and virtual radiation with vanishing momentum [14]. Consequently, for many processes the NLO calculations represent the most accurate theoretical predictions and NNLO (next-to next-to-leading order) calculations are available only for a handful of processes of primary interest to the physics at highenergy colliders. The latter include the Drell-Yan process, mediated by a virtual-photon and the W and Z boson production [21].
|
Table des matières
Contents
Abstract
Contents
Acknowledgements
Preface
1 Luminosity
1.1 Relevance of absolute luminosity measurements
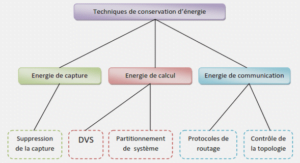
1.1.1 Hard-scattering QCD formalism
1.1.2 Cross-section measurements
1.1.3 Parton distribution functions
1.2 Methods for absolute luminosity determination
1.2.1 Indirect methods
1.2.2 Direct methods
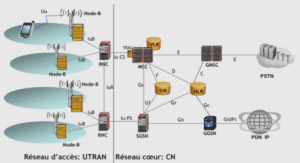
2 The Large Hadron Collider
2.1 CERN and the Large Hadron Collider
2.2 Characteristics and operation
2.2.1 Beam dynamics
2.2.2 Filling scheme
2.2.3 Beam instrumentation
2.2.4 Operational aspects
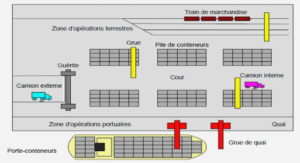
3 The LHCb experiment
3.1 Overview
3.2 Tracking system
3.2.1 Vertex Locator
3.2.2 Tracker Turicensis, Inner Tracker and Outer Tracker
3.2.3 Magnet
3.2.4 Track reconstruction
3.2.5 Primary vertex reconstruction
3.2.6 Performance
3.3 Calorimeters
3.4 Trigger system
3.4.1 LHCb trigger system
3.4.2 Beam gas trigger
3.4.3 Trigger for relative luminosity measurement
3.4.4 Trigger for van der Meer scans
4 Measurement of the relative luminosity
4.1 Luminosity counters
4.2 Methods for the determination of the average number of visible pp interactions per crossing
4.2.1 Average of the counter distribution
4.2.2 Fit to the counter distribution
4.2.3 Zero-count method
4.3 Systematic effects
4.4 Data handling
5 Beam intensity measurement
5.1 Total beam current
5.2 Ghost charge measurement
5.2.1 Event selection
5.2.2 Systematic corrections
5.2.3 Ghost charge measurement in LHC fills 1653 and 1658
5.3 Bunch-by-bunch fractions
5.4 Satellite bunches
5.5 Uncertainties
6 The van der Meer scan method
6.1 Experimental conditions during the van der Meer scans
6.2 Time stability
6.3 Cross-section determination
6.4 Length scale calibration
6.5 Systematic errors
6.5.1 Coupling between the x and y coordinates in the LHC beams
6.5.2 Ghost charge and satellite bunches during the VDM scans
6.5.3 Reproducibility of the luminosity at the nominal beam positions
6.5.4 Cross-check with the z position of the luminous region
6.5.5 Summary of the systematic errors
6.6 Results of the van der Meer scans
7 The beam-gas imaging method
7.1 Data-taking conditions
7.2 Analysis and data selection procedure
![]() Télécharger le rapport complet
Télécharger le rapport complet