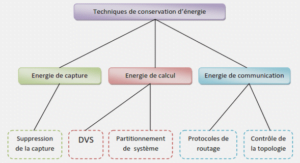
Description du bassin versant du Mackenzie

Description du bassin versant du Mackenzie

Le fleuve Mackenzie, un parmi les plus grands fleuves au monde, draine un bassin d’une superficie totale de 1.8 million de km2 , approximativement 20% de la superficie du Canada (Figure 1 ). Le bassin du Mackenzie se compose de six sous-bassins hydrographiques. Il comprend également trois lacs parmi les plus grands au monde, à savoir, le lac de l’Athabasca, le lac des Esclaves et le lac de l’Ourse. Le delta de l ‘Athabasca qui s’étale sur une superficie approximative de 4 000 km2, à l’ extrémité ouest du lac, est l ‘un des plus grands deltas intérieurs au monde. La végétation au bassin du fleuve Mackenzie est très variable. Elle est plus dense (boréale) au sud du bassin et moins dense (toundra) au nord. Le relief du bassin est également très variable. Les montagnes occupent les limites ouest du bassin. Les régions centrales, à l’est, sont généralement planes. Le débit à l’exutoire du fleuve, qui s’écoule du sud vers le nord, avec une moyenne annuelle de 9 100 m3 /s, est le deuxième au pays de point de vue importance après celui du fleuve Saint-Laurent. Le régime d’écoulement dans le fleuve et les rivières dans le bassin est souvent perturbé pendant la fonte printanière à cause de la formation d’embâcles. Ceci augmente les niveaux d’eau est accentue le risque d’inondation dans le bassin. Cependant, cette importante ressource hydrique est sujette à des fluctuations considérables générées par la variation des conditions climatiques (Shabbar et al., 1997).

L’effet de la variation des conditions climatiques se reflète sur trois paramètres principaux, à savoir, la température, la précipitation et le débit à l’exutoire. En premier lieu, la température saisonnière moyenne dans le bassin varie de -30°C pendant l ‘hiver jusqu’à + 15°C pendant l’ été. Au cours de certains jours particuliers de l’année cette température peut atteindre -50°C en hiver et + 30°C en été présentant ainsi un gradient considérable de 80°C. Cependant, c’est la variation interannuelle de la température qui semble être la plus dramatique pour le bassin. En effet, le Mackenzie présente actuellement un des plus grands taux de réchauffement dans le monde atteignant pendant la saison froide une valeur de 2°C par 10 ans. La non-linéarité de cette augmentation complique encore plus la situation (Stewart et al., 1998). Par ailleurs, la moyenne annuelle de la précipitation dans le bassin du Mackenzie a été estimée de 400 mm/an avec des quantités plus importantes habituellement pendant l’été. Le suivi de l’évolution au cours des années de la précipitation dans le bassin laisse voir également une variation interannuelle. La distribution spatiale de l’intensité moyenne de précipitation, obtenue à partir de l’étude de Stewart et al. ( 1998) est illustrée par la figure 2 et montre des intensités plus élevées dans la région sud-ouest du bassin, avec une moyenne qui peut atteindre 1 000 mm/an. Dans les régions centrales, la précipitation annuelle moyenne est considérablement réduite par les montagnes se trouvant tout au long de la limite ouest du bassin. Ces montagnes, s’élevant de 2 à 3 km d’altitude, jouent le rôle d’obstacles au déplacement de l’humidité provenant de l’océan Pacifique.

Hypothèses de la recherche

L’hypothèse de base de ce travail est la dominance dé l’effet de l’humidité de sol et des plans d’eau tels que les lacs et les rivières dans le signal capté en micro-ondes passives. Il a été supposé également que la sensitivité des micro-ondes passives à l’humidité du sol dépend de l’hétérogénéité des pixels visés par le satellite. Ceci signifie que la considération de l’hétérogénéité est susceptible d’améliorer l’estimation de l’humidité du sol.

Ce travail portera, entre autres, sur la vérification de cette deuxième hypothèse et le filtrage du bruit qui pourrait être introduit par le couvert végétal et/ou la rugosité de la surface. La troisième hypothèse porte sur la variabilité spatiale et temporelle des paramètres caractérisant l ‘état de la végétation et la rugosité de surface dans la région du Mackenzie. La prise en compte de la variabilité dans l’ espace est exigée par l’hétérogénéité et la diversité des modes d’occupation du sol dans le bassin. La variabilité dans le temps est par ailleurs suggérée par l’ évolution rapide au cours de la saison d’été du couvert végétal et des conditions à la surface. Il a été supposé dans cette recherche que cette variabilité temporelle est périodique et qu’elle respecte la même tendance d’une saison à l ‘autre. L’analyse de cette variabilité spatio-temporelle est donc essentielle pour une meilleure estimation de la réserve hydrique dans le Mackenzie. Un des objectifs de ce travail sera de valider cette hypothèse et d’adapter le principe de l’ajustement dynamique des paramètres pour estimer le contenu en eau du sol par un modèle alimenté par des paramètres variables dans l’ espace et dans le temps.

Utilisation du modèle de transfert radiatif pour l’estimation de l’humidité du sol

L’utilisation des fréquences élevées impose une considération de l’effet de l’atmosphère et du couvert végétal qui contribue par ses différentes composantes, feuillage, branches et tiges. Le modèle proposé par Choudhury et al. (1990) a été recommandé pour ces fréquences élevées. Lakshmi (1996) s’est basé principalement sur l’approche de Choudhury et al. (1990) pour la caractérisation du couvert végétal et la modélisation de la diffusion du rayon électromagnétique dans le milieu végétal. Il a également fait appel à un modèle   hydrologique pour simuler l’humidité dans la couche superficielle du sol. Le logiciel utilisé par Lakshmi (1996) est similaire à WATCLASS (Verseghy, 2000). L’approche recommandée par les deux auteurs (Choudhury et al., 1990; Lakshmi, 1996) s’avère exigeante en terme d’informations caractérisant le couvert végétal. Son application dans le cas du Mackenzie nécessite donc une connaissance exacte des caractéristiques du couvert végétal. Par ailleurs, le modèle proposée par Njoku et al. (2003) simplifie la modélisation de l ‘effet de la végétation.

Le modèle a été validé par des mesures SMMR afin de pouvoir l’utiliser avec les données du nouveau capteur AMSR-E. L’apport principal de l’approche suggérée par Njoku et al (2003) réside dans l’estimation d’un vecteur d’inconnu par un processus itératif visant la minimisation de l’écart entre les températures de brillances simulées par le modèle de transfert radiatif et celles observées par le capteur. Njoku et al. (2003) ont utilisé trois fréquences, chacune avec deux polarisations, générant ainsi un vecteur de six variables d’entrée. Dans une autre étude, Bindlish et al. (2003) ont également utilisé la même méthodologie itérative pour estimer un vecteur de trois inconnues à savoir, l’humidité du sol, la température de surface et l’épaisseur optique de la végétation. Bindlish et al.. (2003) ont utilisé une seule fréquence (10,65 GHz) avec deux polarisations en créant un vecteur d’entrée composée par TbH ,TbV et le ratio TbH/TbV. Dans les deux études de (Bindlish et al., 2003; Njoku et al., 2003) la méthode des moindres carrées itératives a été utilisée pour minimiser l’écart entre les variables d’entrée et les résultats des simulations.

La limitation principale de ces deux dernières études réside dans la constance des paramètres du modèle de transfert radiatif. Les paramètres de végétation et de la rugosité mis en jeu dans ces études sont constants et ne varient pas avec l’évolution des conditions à la surface. L’approche de Njoku et al. (Njoku et al., 2003) a été testée sur un site homogène (le désert). Le changement de l’état de la végétation au cours de la saison estivale recommande fortement l’ajustement des paramètres du modèle. Par ailleurs Njoku et al. (2003) ont noté que l’approche a été validée par des paramètres météorologiques. Ils ont recommandé pour une meilleure évaluation de ses performances l’utilisation d’un modèle hydrologique sol-atmosphère similaire à WATCLASS, capable de fournir des estimations indépendantes de l’humidité du sol.

Estimation of the water surface extent using passive microwave data

Basist et al (1998) proposed an approach based on the correlation between the decrea·se of emissivity and the brightness temperature differences. The graduai change in space of the land emissivity depends on the frequency and the amount of water at and/or near the surface. They defined a Basin Wetness Index (BWI), which is sensitive to liquid water within the satellite field of view (FOV). This index was estimated by a linear combination ofbrightness temperatures remotely sensed by SSM/I in the 19, 37 and 85 GHz vertically polarized frequencies.  In addition, these parameters account for the mismatching FOVs, which vary from 60 km at 19 GHz to 15 km at 85 GHz (Basist et al., 2001; Tanaka et al., 2003a). This index allows the calculation of the WSF (Equation 2.b ), which is a potential indicator of water storage within the upper soil layer. Refer to Basist et al. (1998) for a complete description of the procedure to calculate the BWI. According to Basist et al (1998), empirical parameters, j30 and 131. are constant for both space and time. Williams et al (2000) have proposed calibrating them based on surface types. Hence, a preliminary classification exercise was required in their study to identify surface types. Several surface types need to be defined in order to improve the reliability of the approach (Williams et al., 2000).

The temporal evolution of the vegetation and surface conditions should have an effect on the emission process and consequently on the relationship between the BWI and the brightness temperature differences. The vegetation growth during the summer season decreases the portion of soil surface « seen » by a microwave sensor and affects the soil emissivity. However, brightness temperatures are highly affected by the presence of moisture near the soil surface. As a result, the BWI sensitivity to soil moisture should vary during the summer season. Therefore, the relationship between the emissivity reduction, due to wetness, and the brightness temperature differences which define the BWI, needs to be readjusted over time. It is essential to take into account the evolution of the vegetation cover in order to improve the index sensitivity to wet surfaces. In order to overcome the classification step and account for the temporal evolution of the vegetation cover, it is suggested to dynamically update the parameters of the BWI at the reception of each new image and on a pixel per pixel basis. Hence, a mobile window was programmed to scan the image. The window width was fixed at 5 pixels.

Considering the SSM/1 image resolution, the total width of the window is 125 km. Considering that it is the trend of the parameters that will be used to estimate BWI and FWS, the size of the mobile window does not seem to be crucial. At the reception of each new image, the empirical parameters are estimated for the central pixel of the mobile window, allowing the temporal variation of these parameters to be assessed. The results obtained will be used in this study as an estimation of the WSF. It is expected that this dynamic readjustment will improve the BWI, and therefore the WSF sensitivity to the liquid water on the soil surface. SSM/1 data,used in this study, were extracted from the National Snow and lee Data Center (NSIDC) database (Armstrong et al., 1994). The resolution of the SSM/1 imported images (ascending mode) is 25 km for 19 and 37 GHz, and 12.5 km for 85 GHz, which are mapped in polar Azimuthal Equal-Area grids. The 19, 37 and 85 GHz vertically polarized frequency channels of the SSM/1 sensor were used to estimate the WSF by a linear combina ti on of these frequencies.

The rating curve formula

Severa} studies have proven that there is a strong correlation between hydrological nparameters, discharge or stage, and flooded areas (Frazier et al., 2003; Mosley, 1983; Smith et al., 1996; Vorosmarty et al., 1996). Smith et al. (1995) used the measurements of flooded areas derived from ERS-1 SAR images to estimate the discharge of a glacial river in British Columbia.  Despite its simplicity and interesting potential, particularly over large watersheds, the main problem of using this simple empirical relationship in flood forecasting is its dependency on surface type conditions. The empirical parameters vary significantly from one site to another. Moreover, the temporal evolution of the surface conditions in the basin suggests that these parameters may weil vary with time. It is interesting to analyze the potential of this model for water surface extent prediction using passive microwave data and discharge measurements. The problem of spatial and temporal constancy of the classic power law model parameters will be particularly examined. The relationship between flooded areas and discharge in northem climates depends largely on the occurrence of ice jams in the main channel of rivers.

Moreover, the presence of vegetation and semi-active zones that join the hydrological network only during flooding can also control the relationship between discharge and water body extent. Generally, the flooded area increases with increasing discharge . This relationship leads to a rating curve model relating the flooded area to the discharge, written as: WSF(t) =a. Qb (t) (4.4) where WSF is the Water Surface Fraction, Q is the measured discharge, and a and b are two empirical parameters. Generally, these empirical parameters are determined by a linearization of Equation 4.4. The power law model (Equation 4.4) was selected because of its simplicity and the availability of the parameters required for its implementation. The easy availability of the parameters represents a great advantage for estimating the water body extent. The relationship between the flow and the WSF is expected to vary with time. These different data sets will affect the estimation accuracy of the parameters of the rating curve model. Thus, the estimation of the parameters should account for the changing relationship between the flow and the WSF.

Study area: The Mackenzie River Basin

The methodology proposed in this work was applied to the Mackenzie River Basin (MRB). Going from south to north, the vegetation zones in the Mackenzie Basin vary from prairies to boreal forests to tundra, in the northerly part of the basin. In the western part of the basin, mountains rise up to 3 km, and the lowest zones lie in the northern and central-eastern parts of the basin (Rouse, 2000; Stewart et al., 1998). The Mackenzie Basin contains sorne of the largest freshwater lakes in the world: Great Bear Lake, Great Slave Lake and Athabasca Lake. These lakes play an important role in water cycle monitoring in the Mackenzie River Basin. Freshwater lakes and distributary channels co ver up to 50% of the total Mackenzie Delta area (Burn, 1995). The water cycle in the MRB is largely affected by the spring snow melt period, during which ice thawing is its major source of freshwater. Similarly, in the Basin’s lakes and rivers, the water level rises to its highest level during this period. Consequently, river discharge becomes very significant and many lakes flooded. Note that flooding over the Mackenzie River Basin is largely affected by ice jamming. Marsh and Hey ( 1989) stated that 33% of lakes in the Mackenzie Delta can be considered as high-closure lakes. In this case, ice jamming dominates flooding. Thus, these lakes are mainly flooded during the spring melt period, when ice jamming occurs. Over the Peace-Athabasca Delta, Toyra et al. (2002) observed several wetland basins surrounding the delta. The central part of thedelta is mainly composed of large and shallow lakes which are connected to Lake Athabasca by several channels. Generally, these wetland basins are not connected to the main channel. However, following the ice break-up, a portions of these basins flood rapidly (Leconte et al., 2001).

Renee, flooding in two major deltas of the Mackenzie River Basin, namely, the Mackenzie River delta and the Peace-Athabasca Delta, depends largely on the spring snow melt period. Furthermore, flooding frequencies in these areas are highly related to their morphology as most of these wetland basins are disconnected, and only join the main river during the spring melt period as a result of ice jams. Therefore, during the spring melt period, these flooded areas include either basins covered by shallow water or wetlands with highly moist soils.

Approach reliability assessment using 1998 and 1999 data

WSF values over the Mackenzie River Basin were estimated during the summers of 1998 and 1999 on a pixel-per-pixel basis. These estimations were used in this application to carry out offline simulations, and were considered as  »true » values to which the predicted WSF were compared. From the daily generated WSF maps, the Peace Athabasca Delta (P AD) and the Mackenzie River delta (MRD) were selected as control sections in order to assess the reliability of the proposed approach. The PAD and the MRD have areas of about 4000 and 12000 km2, respectively. The estimated WSF was averaged over each control section in order to apply the proposed methodology. The estimated WSF can be affected by atmospheric conditions, especially during wet seasons (Kerr & Njoku, 1993). However, the flooding effect will dominate the WSF variability as simulations were carried out over two deltas with a high flood potential. The existence of a significant correlation between flooded areas and discharges over these areas is the most important reason for choosing these two deltas for the application of the methodology. Furthermore, the flat topography of these selected deltas reduces the contribution to the satellite signal of high-closure lakes that do not join the main river stem, even during the spring melt period. Moreover, previous studies (Sippel et al., 1992; Vorosmarty et al., 1996) recommended an analysis of the correlation between discharge and floodplain extent within the main stem. The main river stems were included in the selected portions in this study.

Table des matières

SOMMAIRE
ABSTRACT
REMERCIEMENTS
LISTE DES FIGURES
LISTE DES ABRÉVIATIONS ET SIGLES
CHAPITRE 1 INTRODUCTION
1.1 Problématique
1.2 Description du bassin versant du Mackenzie
1.3 Objectifs de la thèse
1.4 Hypothèses de la recherche
1.5 Présentation de la thèse
CHAPITRE 2 UTILISATION DES MICRO-ONDES PASSIVES POUR L’ESTIMATION DE L’HUMIDITÉ DU SOL
2.1 Justification du choix des micro-ondes passives
2.2 Estimation de l’humidité du sol par les micro-ondes passives: État de connaissance
2.3 Les principaux facteurs affectant l’estimation de l’humidité du sol
2.3.1 La rugosité de surface
2.3.2 La végétation
2.3.3 Effet atmosphérique
2.4 Discussion
2.4.1 Approches globales pour l’estimation de l’humidité du sol
2.4.2 Utilisation du modèle de transfert radiatif pour 1′ estimation de 1 ‘humidité du sol
CHAPITRE 3 A DYNAMIC ESTIMATION OF FREE WATER SURF ACE COVERAGE FROM A BASIN WETNESS INDEX OF THE MACKENZIE RIVER BASIN USING SSM/I MEASUREMENTS
3.1 Introduction
3.2 The Basin Wetness Index
3.3 Application
3.4 Conclusion
CHAPITRE 4 FLOOD MONITORING OVER THE MACKENZIE RIVER BASIN USING PASSNE MICROWAVE DATA 
4.1 Introduction
4.2 Methodology
4.2.1 Estimation of the water surface extent using passive microwave data
4.2.2 The rating curve formula
4.2.3 Use of the of Kalman filter
4.3 Application and Results
4.3.1 Study area: The Mackenzie River Basin
4.3.2  Approach reliability assessment using 1998 and 1999 data
4.3.3 Use of passive microwave data for discharge forecasting
4.4 Conclusion 
CHAPITRE 5 FLOOD AND SOIL WETNESS MONITORING OVER THE MACKENZIE RNER BASIN USING AMSR-E 37 GHZ BRIGHTNESS TEMPERA TURE
5.1  Introduction 
5.2 Methodology
5.2.1 Definition of the Wetness Index
5.2.2  The linear mixing model
5.2.3 Calibration of the mixing model parameters
5.2.4 Development of the Ra ting Curve Model
5.3 Results and Discussion
5.4 Conclusion 
CHAPITRE 6 A COMBINATION OF REMOTE SENSING DATA AND TOPOGRAPHie ATTRIBUTES FOR THE SPATIAL AND TEMPORAL CONTROL OF THE SOIL WETNESS OVER THE MACKENZIE RNER BASIN
6.1 Introduction
6.2 Methodology
6.2.1 Area of study
6.2.2 The wetness index
6.2.3 Estimation of the basin wetness index, BWI, using passive microwave data
6.3 Results and discussion
6.3.1 Development of the WI maps
6.3.2 Estimation of the BWI
6.3.3 Combination of the WI and the BWI
6.4 Conclusion
CHAPITRE 7 ANALYSE ET DISCUSSION
7.1 Contribution de la thèse
7.2 Recommandations et travaux futurs
CONCLUSION
Tableau 1.
Tableau II
BIBLIOGRAPHIE

Télécharger le rapport complet